Comments on Puzzle #31492: A puzzle I saw in my childhood
By Ron Jacobson (shmily999)
peek at solution solve puzzle
quality: 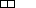 difficulty:
difficulty:  solvability: line logic only
solvability: line logic only
peek at solution solve puzzle
quality: 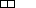 difficulty:
difficulty:  solvability: line logic only
solvability: line logic only
Puzzle Description Suppressed:Click below to view spoilers
#1: Ron Jacobson (shmily999) on Aug 22, 2018
This is a puzzle I saw in the newspaper when I was in middle school. An easy task... you were asked to draw a continuous line that crossed each line segment of the picture.#2: Dik Hz (dikhz) on Aug 22, 2018 [SPOILER]
After a while of trying, I learned it was impossible, but to this day, I still will draw this figure and try to do it.
What does that say about me? Hmmmmmm
Comment Suppressed:Click below to view spoilers#3: Brian Bellis (mootpoint) on Aug 22, 2018
Roll the paper into a cylinder around a suitable diameter tube and draw a single diagonal straight line until it passes through each line segment.#4: Alan Lafond (Cural) on Aug 22, 2018 [SPOILER]
Comment Suppressed:Click below to view spoilers#5: Alan Lafond (Cural) on Aug 22, 2018 [HINT] [SPOILER]
Comment Suppressed:Click below to view hints and spoilers#6: Brian Bellis (mootpoint) on Aug 23, 2018
Oh, that kind of "line".#7: Kerri Kemp (kerri_kemp) on Aug 23, 2018 [SPOILER]
You have to cross each line segment only once.
Comment Suppressed:Click below to view spoilers#8: Ron Jacobson (shmily999) on Aug 23, 2018
Yes, Cural, it is. Can't go through the corners.#9: Alan Lafond (Cural) on Aug 24, 2018 [HINT] [SPOILER]
Comment Suppressed:Click below to view hints and spoilers#10: John Macdonald (perlwolf) on Jan 4, 2019
Rather than 3 odd-sided rooms, it is 4 odd-sided areas (with the courtyard surrounding the building as the additional area that has an odd number of sides. (Each wall segment must be a border of two areas, so the total number of border segments is even - so there must be an even number of areas than have an odd number of border segments.)
An area with an odd number of border segments can only be the first or last room visited. Whenever your path goes through an area, you remove 2 segments from its unvisited list. So, if a room has an odd number of border segments and you do not start there, then every time you go through that area you remove two of the segments until there is only one left. After that, you cannot go through the room again - if you go through the last segment you must remain in that area, so this must be the last area visited. If you start in an odd-sided area, then you remove one segment when you leave, so there is an even number of segments remaining, so you can go through that area enough times to use up the remaining even number of segments (and leave after the last one is crossed). If you start in an even-sided area, then after you leave it has an odd number left and you must end in that area just like an odd-sided area that you didn't start in. So, you can solve a puzzle in two ways:
If all of the areas have an even number of segments, start anywhere and end in that same area,
If there are two odd-sided areas, start in one of them and end in the other.
If there are more than two odd-sided areas, then that puzzle cannot be solved since the start and end can only provide a fix for two odd-sided areas.
You must register and log in to be able to participate in this discussion.